1 Answer

Alexandru Dinu · Answer 1 · 2023-10-16T13:27:33+0000

- - - - - - - - - - - - - - - - - - - - - - -- - - - - - - - - -

$\large\blue\textsf{\textbf{\underline{\underline{Question:-}}}}$

What kind of number is
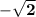$\bold{-√(2)}$ ?

$\large\blue\textsf{\textbf{\underline{\underline{Answer and How to Solve:-}}}}$

First, let's look at each of the four sets of numbers:-

Rational. This number set includes natural numbers (positive numbers only, like 1, 2, 3, 4, 5, 6...), zero, integers (negative & positive numbers and zero) and fractions (1/2, 1/3, 1/4...)
Irrational. This set includes numbers that have an infinite quantity of digits after the decimal point. Also, irrational numbers cannot be written as fractions.
Not a real number. This set includes imaginary numbers (yes, such numbers exist in mathematics, believe it or not)
Natural number. As I said earlier, this set includes positive numbers only.

So in which set does
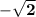$\bold{-√(2)}$ belong?

First, it's negative, so it's not a natural number.

Then, it's not an imaginary number, so it doesn't belong in the "Not a real number" set.

It can't be written as a fraction, so it doesn't belong in the "Rational" set, but it does belong in the "Irrational numbers" set.

According to the answer and explanation, we conclude that the number
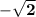$\bold{-√(2)}$ belongs in the "Irrational number" set.

- - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - - -

Please log in or register to add a comment.