Answer:
18 square units.
Explanation:
Area of the triangle = ½*AB*CD
First of all, find the length of AB and CD using the distance formula,
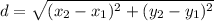
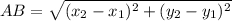
A(-6, 2) => (x1, y1)
B(3, 2) => (x2, y2)
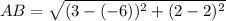
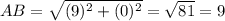
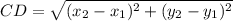
C(-2, 6) => (x1, y1)
D(-2, 2) => (x2, y2)
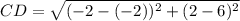
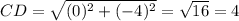
AB = 9
CD = 4
Area of rectangle = ½*AB*CD = ½*9*4 = 9*2 = 18 square units.