Answer:
The value is
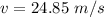
Yes
Step-by-step explanation:
From the question we are told that
The mass of the car is

The radius is
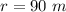
The coefficient of static friction is
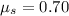
Generally at maximum speed the centripetal force acting on the car is equal to the friction force on the car
So
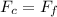
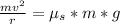
=>
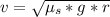
=>
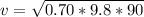
=>
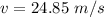
Yes the value is independent of the mass because from the equation above we see that v is independent of mass