Question

Find the values for k so that the intersection of x=2k and 3x+2y=12 lies in the first quadrant.

Answer 1

Values of k can be 0, 1, or 2 such that intersection of the given lines lie in the 1st quadrant.

Explanation:

Given two lines:

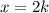`x=2k` and

`3x+2y=12`

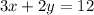
To find:

Values of 'k' such that the intersection of given two lines lie in the first quadrant.

Solution:

In 1st quadrant, the values of
`x` and
`y` both are positive.

So, let us find out intersection of the two lines.

Intersection of the two lines can be found by solving the two equations for the values of
`x` and
`y`.

Given that
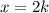`x=2k` to be in the first quadrant, the value of k must be positive.

Let us put
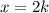`x=2k` in the equation
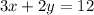`3x+2y=12` to find the intersection point.

`3 * 2k + 2y=12\\\Rightarrow 6k+2y=12\\\Rightarrow 2y=12-6k\\\Rightarrow \bold{y=6-3k}`

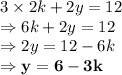
For y to be positive:

`6 - 3k \geq 0\\\Rightarrow 3k \leq 6\\\Rightarrow k \leq 2`

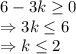
So, values of k can be 0, 1, or 2 such that intersection of the given lines lie in the 1st quadrant.