Answer:
The torque torque produced on the turbine from the wind is approximately 955 kN·m
Step-by-step explanation:
The number of revolution per minute of the turbine = 20 rev/min
The power output of the turbine = 2.0 MW
The power transmitted to a shaft equation is given as follows;
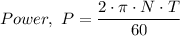
Where;
P = The power transmitted to a turbine shaft = 2.0 MW
N = The number or revolutions per minute = 20 rev/min
T = The torque produced on the turbine by the wind
Therefore;

The torque torque produced on the turbine from the wind = 3/π MN·m ≈ 0.955 MN·m = 955 kN·m.