Question

48% of their readers own a particular make of car. A marketing executive wants to test the claim that the percentage is actually different from the reported percentage. A random sample of 300 found that 45% of the readers owned a particular make of car. Is there sufficient evidence at the 0.02 level to support the executive's claim?

Answer 1

There is no sufficient evidence to support the executive claim

Explanation:

From the question we are told that

The population proportion is
`p = 0.48`

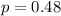
The sample proportion is
`\r p = 0.45`

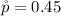
The sample size is
`n = 300`

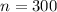
The level of significance is
`\alpha = 0.02`

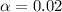
The null hypothesis is
`H_o : p= 0.48`

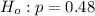
The alternative hypothesis is
`H_a : p \\e 0.48`

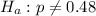
Generally the test statistics is mathematically evaluated as

`t = \frac{\r p - p }{ \sqrt{ (p(1 - p ))/(n) } }`

=>
`t = \frac{0.45 - 0.48 }{ \sqrt{ (0.48 (1 - 0.48 ))/(300) } }`

=>
`t = -1.04`

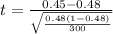
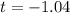
The p-value is mathematically represented as

`p-value = 2P(z > |-1.04|)`

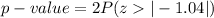
Form the z-table

`P(z > |-1.04|) = 0.15`

=>
`p-value = 2 * 0.15`

=>
`p-value = 0.3`

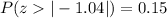
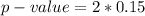
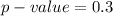
Given that
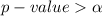`p-value > \alpha` we fail to reject the null hypothesis

Hence we can conclude that there is no sufficient evidence to support the executive claim