Question

A city has a population of people. Suppose that each year the population grows by . What will the population be after years?

Answer 1

The question is missing the values, I found a possible matching question:

a city has a population of 380,000 people. suppose that each year the population grows by 7.5%. what will be the population after 6 years

Answer:

After 6 years, the population will be 586, 455 people

Explanation:

This growth is similar to the growth of an invested amount of money, which is compounded annually, yielding a future value, when it increases by a certain interest rate. Hence the formula for compound interest is used to determine the population after 6 years as follows:

`FV = PV (1+ (r)/(n))^({n * t})`

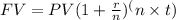
where

FV = future value = population after 6 years = ???

PV = present value = current population = 380,000 people

r = interest rate = growth rate = 7.5% = 7.5/100 = 0.075

n = number of compounding periods per year = annually = 1

t = time of growth = 6 years

`FV = 380,000 (1+ (0.075)/(1))^({1 * 6})\\FV = 380,000 (1.075)^(6)\\FV= 380,000 (1.5433015256)\\FV = 586,454.58\\FV= 586,455\ people`

Therefore, after 6 years, the population will be 586, 455 people