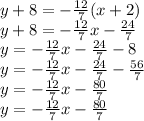Answer:
Explanation:
In order to find the slope-intercept form of a line when given two points, we need to first put it into point-slope form. The point-slope form is given by the equation:

Where m is the slope and x₁ and y₁ is one of the two points.
Anyways, let's first find the slope. Let's designate (-2,-8) as x₁ and y₁ and (-9,4) as x₂ and y₂ (it doesn't really matter). The formula for slope is:
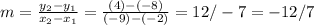
So, the slope is -12/7.
Now, pick a point for the point-slope form. To keep things consistent, I'm going to use the point (-2,-8) as x₁ and y₁. Plug in -12/7 for m. Therefore:
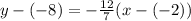
Now, simplify, distribute, and isolate the y: