Question

Find the equation for the line parallel to y=x+3 which has the same y intercept as 2x+3y=12

Answer 1

`y=x+4`

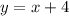
Explanation:

Two parallel lines will have the same slope:

`y=mx+b`

This is the y-intercept equation, where m is the slope and b is the y-intercept:

`y=x+3`

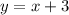
The slope is x, or 1x, so the parallel line is also x.

Find the y-intercept:

You do this by taking the equation given and solving for y:

`2x+3y=12`

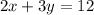
Isolate the variable y. Subtract 2x from both sides:

`2x-2x+3y=12-2x\\\\3y=12-2x`

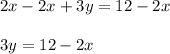
Isolate y by dividing both sides by 3:

`(3y)/(3)=(12)/(3)-(2x)/(3) \\\\y=4-(2)/(3)x`

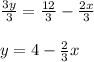
Rearrange in slope-intercept form:

`y=-(2)/(3)x+ 4`

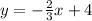
The y-intercept of this equation is 4.

Now make the equation using the given information:

`y=x+4`

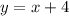
:Done