Answer:
(a) the probability that an individual distance is greater than 214.80 cm is 0.1401.
(b) The probability that the mean for 15 randomly selected distances is greater than 204.00 cm is 0.2482.
(c) The normal distribution can be used because the original population has a normal distribution.
Step-by-step explanation:
We are given that the overhead reach distances of adult females are normally distributed with a mean of 205.5 cm and a standard deviation of 8.6 cm.
(a) Let X = the overhead reach distances of adult females.
The z-score probability distribution for the normal distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean reach distance = 205.5 cm
= population mean reach distance = 205.5 cm
 = standard deviation = 8.6 cm
= standard deviation = 8.6 cm
So, X ~ Normal(
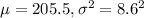 )
)
Now, the probability that an individual distance is greater than 214.80 cm is given by = P(X > 214.80 cm)
P(X > 214.80 cm) = P(
 >
>
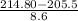 ) = P(Z > 1.08) = 1 - P(Z
) = P(Z > 1.08) = 1 - P(Z
 1.08)
1.08)
= 1 - 0.8599 = 0.1401
The above probability is calculated by looking at the value of x = 1.08 in the z table which has an area of 0.8599.
(b) Let
 = the sample mean selected distances.
= the sample mean selected distances.
The z-score probability distribution for the sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean reach distance = 205.5 cm
= population mean reach distance = 205.5 cm
 = standard deviation = 8.6 cm
= standard deviation = 8.6 cm
n = sample size = 15
Now, the probability that the mean for 15 randomly selected distances is greater than 204.00 cm is given by = P(
 > 204.00 cm)
> 204.00 cm)
P(
 > 204 cm) = P(
> 204 cm) = P(
 >
>
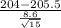 ) = P(Z > -0.68) = 1 - P(Z
) = P(Z > -0.68) = 1 - P(Z
 0.68)
0.68)
= 1 - 0.7518 = 0.2482
The above probability is calculated by looking at the value of x = 0.68 in the z table which has an area of 0.7518.
(c) The normal distribution can be used in part (b), even though the sample size does not exceed 30 because the original population has a normal distribution and the sample of 15 randomly selected distances has been taken from the population itself.