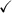Answer: see proof below
Explanation:
Use the following identities
tan (A + B) = (tan A + tan B)/(1 - tan A · tan B) -->

tan 60° = √3
tan 120° = -√3
tan 3A = (3tanA - tan³A)/(1 - 3 tan²A) -->
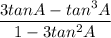
Proof LHS → RHS
Given: tan Ф + tan(60° + Ф) + tan(120° + Ф)
Sum Difference: tan Ф + (tan 60° + tanФ)/(1-tan60°·tanФ) + (tan 120° + tanФ)/(1-tan120°·tanФ)
(latex)
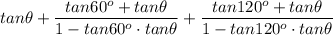
Substitute: tan Ф + (√3 + tanФ)/(1-√3·tanФ) + (-√3 + tanФ)/(1+√3°·tanФ)
(latex)
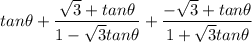
Common Denominator: [tan Ф(1-3tan²Ф)+8tanФ]\(1-3tan²Ф)
(latex)
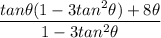
Distribute: (tan Ф - 3tan³Ф + 8Ф)\(1 - 3 tan²Ф)
(latex)
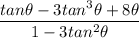
Simplify: (9Ф - 3tan³Ф)\(1 - 3 tan²Ф)
3(3Ф - tan³Ф)\(1 - 3 tan²Ф)
(latex)
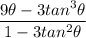
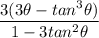
Triple Angle Identity: 3 tan 3Ф
3 tan 3Ф = 3 tan 3Ф