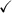Question

I need help to solve this please help me...​

Answer 1

Your question has been heard loud and clear/

we know,

4cos³x = 3cosx + cos3x

put here, x = 20°

then, 4cos³20° = 3cos20° + cos3 × 20°

= 3cos20° + cos60°

cos³20° = (3cos20° + cos60°)/4 -------(1)

similarly ,

4sin³x = 3sinx - sin3x

put here, x = 10°

4sin³10° = 3sin10° - sin30°

sin³10° = (3sin10° - sin30°)/4 -----------(2)

now,

LHS = cos³20° + sin³10°

put equations (1) and (2)

= 1/4(3cos20° + cos60°) + 1/4 ( 3sin10° - sin30°)

= 1/4( 3cos20° + cos60° + 3sin10° - sin30°)

we know,

cos60° = sin30° = 1/2

= 1/4 ( 3cos20° + 3sin10°)

= 3/4(cos20° + sin10°) = RHS

hence proved

Thank you

Answer 2

Answer: see proof below

Explanation:

Use the following identities & Unit Circle calculations:

4cos³x = 3cos x + cos 3x

--> cos³x = (1/4)[3cos x + cos 3x]

4sin³x = 3sin x - sin 3x

--> sin³x = (1/4)[3sin x - sin 3x]

cos 60° = sin 30° = (1/2)

Proof: LHS → RHS

Given: cos³ 20° + sin³ 10°

Triple Angle Identity: (1/4)[3cos 20° + cos 3·20°] + (1/4)[3sin 10° - sin 3·10°]

Simplify: (1/4)[3cos 20° + cos 60° + 3sin 10° - sin 30°]

(1/4)[3cos 20° + (1/2) + 3sin 10° - (1/2)]

(1/4)[3cos 20° + 3sin 10°]

Factor: (3/4)[cos 20° + sin 10°]

Proven: (3/4)[cos 20° + sin 10°] = (3/4)[cos 20° + sin 10°]
`\checkmark`