Question

A mass m = 0.3 kg is released from rest at the origin point 0. The mass falls under the influence of gravity. When the mass reaches point A, it has a velocity of v downward and when the mass reaches point B its velocity is 5v. What is the distance between points A & B divided by the distance between points 0 & A?

Answer 1

24

Step-by-step explanation:

The mass = 3 kg

at point O all the mechanical energy of the system is due to its potential energy PE. The body is at rest.

PE = mgh

but ME = PE + KE = constant (law of energy conservation)

KE is the kinetic energy

since KE is zero at this point, then,

ME = mgh

where m is the mass

g is acceleration due to gravity = 9.81 m/s^2

h is the height = O

ME = 3 x 9.81 x O

ME = 29.43-O

At point A the total ME is due to its PE and its kE

PE at this point = mgh = 3 x 9.81 x A = 29.43-A

KE =
`(1)/(2)mv^(2)`

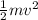
velocity = v at this point, therefore,

KE =
`(1)/(2)*3*v^(2)` =
`(3)/(2) }v^(2)`

therefore,

ME = 29.43-A +
`(3)/(2) }v^(2)`

Equating ME for the points O and A, we have

29.43-O = 29.43-A +
`(3)/(2) }v^(2)`

29.43-O - 29.43-A =
`(3)/(2) }v^(2)`

(O - A)29.43 =
`(3)/(2) }v^(2)`

O - A = 0.051
`v^(2)` this is the distance between point O and A

For point B

PE = 29.43-B

KE =
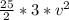`(25)/(2)*3*v^(2)` = 37.5
`v^(2)` (velocity is equal to
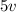`5v` at this point)

therefore,

ME = 29.43-B + 37.5
`v^(2)`

Equating the ME for points A and B, we have

29.43-A +
`(3)/(2) }v^(2)` = 29.43-B + 37.5
`v^(2)`

29.43-A - 29.43-B = 37.5
`v^(2)` -
`(3)/(2) }v^(2)`

(A - B)29.43 = 36
`v^(2)`

A - B = 1.22
`v^(2)` this is the distance between points A and B

The distance between points A & B divided by the distance between points 0 & A will be

1.22
`v^(2)`/0.051 = 23.9 ≅ 24