Answer:
352 seconds are needed for the radioisotope to decay to one-sixteenth of its original mass.
Step-by-step explanation:
The decay of radioisotopes are represented by the following ordinary differential equation:
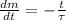
Where:
 - Time, measured in seconds.
- Time, measured in seconds.
 - Time constant, measured in seconds.
- Time constant, measured in seconds.
 - Mass of the radioisotope, measured in grams.
- Mass of the radioisotope, measured in grams.
The solution of this expression is:
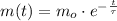
Where
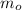 is the initial mass of the radioisotope, measured in kilograms.
is the initial mass of the radioisotope, measured in kilograms.
The ratio of current mass to initial mass is:
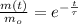
The time constant is now calculated in terms of half-life:
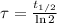
Where
 is the half-life of the radioisotope, measured in seconds.
is the half-life of the radioisotope, measured in seconds.
Given that
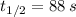 , the time constant of the radioisotope is:
, the time constant of the radioisotope is:
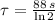

Now, if
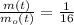 and
and
 , the time is:
, the time is:
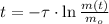
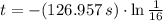
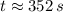
352 seconds are needed for the radioisotope to decay to one-sixteenth of its original mass.