Answer:
The magnitude of the charge is 54.9 nC.
Step-by-step explanation:
The charge on each bead can be found using Coulomb's law:
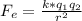
Where:
q₁ and q₂ are the charges, q₁ = q₂
r: is the distance of spring stretching = 4.8x10⁻² m
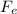 : is the electrostatic force
: is the electrostatic force
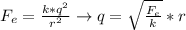
Now, we need to find
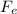 . To do that we have that Fe is equal to the spring force (
. To do that we have that Fe is equal to the spring force (
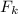 ):
):
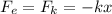
Where:
k is the spring constant
x is the distance of the spring = 4.8 - 4.0 = 0.8 cm
The spring constant can be found by equaling the sping force and the weight force:
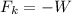
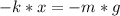
where x is 5.2 - 4.0 = 1.2 cm, m = 1.8 g and g = 9.81 m/s²
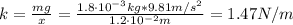
Now, we can find the electrostatic force:
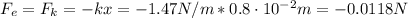
And with the magnitude of the electrostatic force we can find the charge:
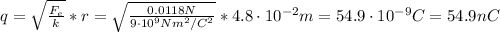
Therefore, the magnitude of the charge is 54.9 nC.
I hope it helps you!