Question

PLEASE HELP SOON! A 2011 study by the National Safety Council estimated that there are nearly 5.7 million traffic accidents year. At least 28% of them involved distracted drivers using a cell phones or texting. The data showed that 11% of drivers at any time are using cell phones. Car insurance companies base their policy rates on accident data that shows drivers have collisions approximately once every 19 years. That's a 5.26% chance per per year. Given.

A - Let dc= event that a randomly selected driver is using a cell phone. what is P(DC)? B - Let ta = event that a randomly selected driver has a traffic accident. what is P(ta) C - how can you determine if cell phone use while driving and traffic accidents are related? D - Give that the driver has an accident, what is the probability that the driver was distracted by a cell phone? Write this event with the correct conditional notation.

Answer 1

(A) 0.11

(B) 0.0526

(C) Related

(D) 0.28

Explanation:

The data provided is:

DC = event that a randomly selected driver is using a cell phone

TA = event that a randomly selected driver has a traffic accident

(A)

From the provided data:

P (DC) = 0.11

(B)

From the provided data:

P (TA) = 0.0526

(C)

To determine whether the events DC and TA are dependent, we need to show that:

`P(DC\cap TA)\\eq P(DC)* P(TA)`

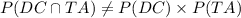
The value of P (DC ∩ TA) is,

`P(DC\cap TA)=P(DC|TA)\time P(TA)`

`=0.28* 0.0526\\=0.014728`

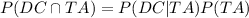
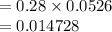
Now compute the value of P (DC) × P (TA) as follows:

`P (DC) * P (TA)=0.11* 0.0526=0.005786`

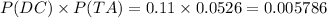
So,
`P(DC\cap TA)\\eq P(DC)* P(TA)`

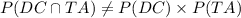
Thus, cell phone use while driving and traffic accidents are related.

(D)

The probability that the driver was distracted by a cell phone given that the driver has an accident is:

P (DC | TA) = 0.28