Question

savanah solved the equation 3+4 multiplied by the absolute value of x/2+3=11 for one solution. her work is shown below. what is the other solution to the given absolute value equation: savanah's solution was x= -2

Answer 1

-10

Explanation:

Given the equation solved by savanah expressed as
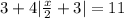`3+4|(x)/(2) + 3| = 11`, IF she solved for one of the solution and got x = -2, we are to solve for the other value of x.

Note that the expression in modulus can be expressed as a positive expression and negative expression.

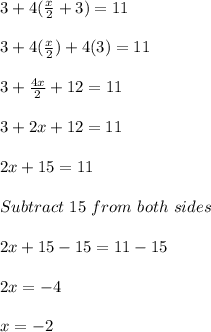
For the positive value of the expression
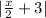`|(x)/(2) + 3|` i.e
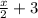`(x)/(2) + 3`, the expression becomes;

`3+4((x)/(2) + 3) = 11`

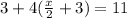
On simplification;

`3+4((x)/(2) + 3) = 11\\\\3 + 4((x)/(2) )+4(3) = 11\\\\3 + (4x)/(2)+ 12 = 11\\\\3 + 2x+12 = 11\\\\2x+15 = 11\\\\Subtract \ 15 \ from \ both \ sides\\\\2x+15-15 = 11-15\\\\2x = -4\\\\x = -2`

For the negative value of the expression
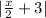`|(x)/(2) + 3|` i.e
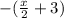`-((x)/(2) + 3)`, the expression becomes;

`3+4[-((x)/(2) + 3)] = 11`

On simplifying;

`3+4[-((x)/(2) + 3)] = 11\\\\3+4(-(x)/(2) - 3)= 11\\\\3-4((x)/(2)) -12 = 11\\\\3 - (4x)/(2) - 12 = 11\\\\3 - 2x-12 = 11\\\\-2x-9 = 11\\\\add \ 9 \ to \ both \ sides\\\\-2x-9+9 = 11+9\\-2x = 20\\\\x = -20/2\\\\x = -10`

Hence her other solution of x is -10