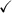Answer: see proofs below
Explanation:
Proofs are like a puzzle that you have to unravel.
You can manipulate the left side to get the right side (LHS → RHS)
or
You can manipulate the right side to get the left side (RHS → LHS)
You will need to use Pythagorean Identities, Sum and Difference Identities, and/or Double Angle Identities when manipulating one of the sides.
Use the following Identities:
cos 2A = cos²A - sin²A
sin 2A = 2 cosA · sinA Note that A can be substituted with 2A
sin 3A = 3 sinA - 4sin³A
cos 3A = 4cos³A - 3cosA
***********************************************************************************
e) Prove: 4 sin A · cos³A - 4 cos A · sin³A = sin(4A)
LHS → RHS
Given: 4 sin A · cos³A - 4 cos A · sin³A
Factor: 4 sin A · cos x (cos²A - sin²A)
2(2 sin A · cos A)(cos²A - sin²A)
Double Angle Identity: 2(sin 2·A)(cos 2·A)
2 sin (2A) · cos (2A)
Double Angle Identity: sin (2·2A)
sin 4A
Proven: sin 4A = sin 4A
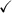
*****************************************************************************************
f) Prove: 4 sin³A · cos 3A + 4 cos³A · sin 3A = 3 sin(4A)
LHS → RHS
Given: 4 sin³A · cos 3A + 4 cos³A · sin 3A
Triple Angle Identity: 4sin³A (4cos³A - 3 cosA) + 4cos³A (3sinA - 4sin³A)
Expand: 16sin³A · cos³A - 12sin³A · cosA + 12cos³A · sinA - 16sin³A · cos³A
Simplify: 12cos³A · sinA - 12sin³A · cosA
Expand: 6cos²A(2cosA · sinA) - 6sin²A(2cosA · sinA)
Double Angle Identity: 6cos²A(sin 2A) - 6sin²A(sin 2A)
Factor: 6sin 2A (cos²A - sin²A)
Double Angle Identity: 6sin 2A (cos 2A)
3(2sin 2A · cos 2A)
Simplify: 3sin (2·2A)
3 sin 4A
Proven: 3sin 4A = 3sin4A