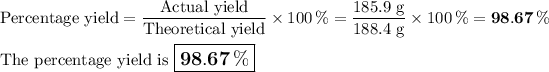Answer:
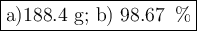
Step-by-step explanation:
We will need a balanced chemical equation with masses and molar masses, so, let's gather all the information in one place.
Mᵣ: 98.08 392.18
2Cr + 3H₂SO₄ ⟶ Cr₂(SO₄)₃ + 3H₂
To solve the stoichiometry problem, you must
- Use the molar mass of H₂SO₄ to convert the mass of H₂SO₄ to moles of H₂SO₄
- Use the molar ratio to convert moles of H₂SO₄ to moles of Cr₂(SO₄)₃
- Use the molar mass of Cr₂(SO₄)₃ to convert moles of Cr₂(SO₄)₃ to mass of Cr₂(SO₄)₃
a) Mass of Cr₂(SO₄)₃
(i) Mass of pure H₂SO₄
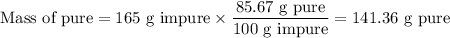
(ii) Moles of H₂SO₄
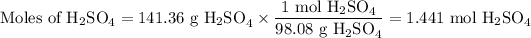
(iii) Moles of Cr₂(SO₄)₃
The molar ratio is 1 mol Cr₂(SO₄)₃:3 mol H₂SO₄

(iv) Mass of Cr₂(SO₄)₃

b) Percentage yield
It is impossible to get a yield of 485.9 g. I will assume you meant 185.9 g.