Question

Find the coordinates of point Q that lies along the directed line segment from R(-2, 4) to S(18, -6) and partitions the segment in the ratio of 3:7.

Answer 1

The coordinates of the point Q is (4, 1)

Explanation:

The given parameters are;

The directed line segment extends from R(-2, 4), to S(18, -6)

The ratio in which the point Q partitions the directed line segment = 3:7

Therefore, the proportions of the R to Q = 3/(3 + 7) = 3/10 the length of RS

Which gives;

(-2 + (18-(-2))×3/10, 4 +(-6 -4)×3/10) which is (4, 1)

The coordinates of the point Q = (4, 1)

We check the length from R to S is given by the relation for length as follows

`l =\sqrt{\left (y_(2)-y_(1) \right )^(2)+\left (x_(2)-x_(1) \right )^(2)}`

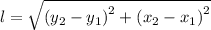
Where;

R(-2, 4) = (x₁, y₁)

S(18, -6) = (x₂, y₂)

Length of segment RS = 22.36

length from R to Q = 6.7086

We check RQ/RS = 6.7082/22.36 = 0.3

Also QS/RS = (22.36 - 6.7082)/22.36 = 0.6999≈ 0.7

The coordinates of the point Q = (4, 1).