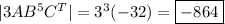Question

Please help me how to do no 5

Answer 1

-864

Explanation:

The determinant of a matrix product is the product of the determinants. The determinant of a transpose is the same as the determinant of the original. Hence ...

`|AB^5C^T|=(4)(-2)^5((1)/(4))=-32`

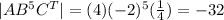
The multiplication of an n×n matrix by a scalar 'a' multiplies its determinant by a^n, so the desired determinant is ...

`|3AB^5C^T|=3^3(-32) = \boxed{-864}`