Question

A basin is filled by two pipes in 12 minutes and 16 minutes respectively. Due to the obstruction of water flow after the two pipes have been running together for some time, the 1st pipe carries 7/8 of the carrying capacity and the 2nd pipe carries 5/6 of the carrying capacity. After removing the water barrier, the tank is full in 3 minutes. How long after the flow of water in the two pipes became normal?​

Answer 1

hope the answer above helped bro! it helped me!

Answer 2

The time duration of the two pipes restricted flow before the flow became normal is 4.5 minutes

Explanation:

The given information are;

The time duration for the volume, V, of the basin to be filled by one of the pipe, A, = 12 minutes

The time duration for the volume, V, of the basin to be filled by the other pipe, B, = 16 minutes

Therefore, the flow rate of pipe A = V/12

The flow rate of pipe B = V/16

Due to the restriction, we have;

The proportion of its carrying capacity the first pipe, A, carries = 7/8 of the carrying capacity

The proportion of its carrying capacity the second pipe, B, carries = 5/6 of the carrying capacity

Whereby the tank is filled 3 minutes after the restriction is removed, we have;

`(7)/(8) * (V)/(12) * t + (5)/(6) * (V)/(16) * t + (V)/(12) * 3 + (V)/(16) * 3 = V`

Simplifying gives;

`((2\cdot t +7) \cdot V)/(16) = V`

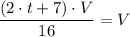
2·t + 7 = 16

t = (16 - 7)/2 = 4.5 minutes

Therefore, it took 4.5 seconds of the restricted flow before the the flow of water in the two pipes became normal