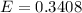Question

A researcher surveys middle-school students on their study habits. She finds that in a random sample of 28 middle-school students, the mean amount of time that they spend working on the computer each night is 2.4 hours with a standard deviation of 0.92 hours. She uses the sample statistics to compute a 95% confidence interval for the population mean - the the mean amount of time that all middle-school students spend working on the computer each night. What is the margin of error for this confidence interval

Answer 1

The margin of error is
`E = 1.96 * ( 0.92)/(√(28 ) )`

Explanation:

From the question we are told that

The sample size is
`n = 28`

The sample mean is
`\= x = 2.4 \ hr`

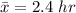
The standard deviation is
`\sigma = 0.92 \ hr`

Given that the confidence level is 95% the the level of significance can be evaluated as

`\alpha = 100 -95`

`\alpha = 5 \%`

`\alpha = 0.05`

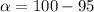
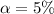
Next we obtain the critical value of
`(\alpha )/(2)` from the normal distribution table,the value is
`Z_{(\alpha )/(2) } = Z_{(0.05)/(2) } = 1.96`

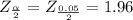
Generally the margin of error is mathematically represented as

`E = Z_{(\alpha )/(2) } * (\sigma )/(√(n) )`

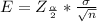
substituting values

`E = 1.96 * ( 0.92)/(√(28 ) )`

`E = 0.3408`