Answer:
The test is a two -tailed test
Explanation:
From the question we are told that
The sample size is n = 31
The sample mean is
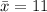
The sample standard deviation is
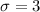
The null hypothesis is
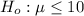
The alternative hypothesis is
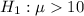
The level of significance is

The test statistics is mathematically represented as

substituting values
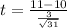
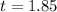
The p- value is mathematically represented as
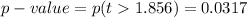
Looking at the value of
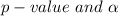 we see that
we see that
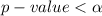 hence we reject the null hypothesis
hence we reject the null hypothesis
Given the that the p value is less than 0.05 it mean the this is a two-tailed test