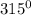Answer:
ii. 75 steps
iii. 75 steps
iv. 106 steps, and
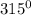
Explanation:
Let Musah's starting point be A, his waiting point after taking 50 steps northward and 25 steps westward be B, and his stopping point be C.
ii. From the second attachment, Musah's distance due west from A to C (AD) can be determined as;
bearing at B =
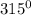 , therefore <BCD =
, therefore <BCD =
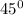
To determine distance AB,
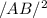 =
=
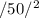 +
+
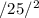
= 25000 + 625
= 3125
AB =
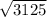
= 55.90
AB ≅ 56 steps
Thus, AC = 50 steps + 56 steps
= 106 steps
From ΔACD,
Sin
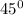 =
=
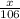
⇒ x = 106 × Sin
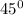
= 74.9533
≅ 75 steps
Musah's distance west from centre to final point is 75 steps
iii. From the secon attachment, Musah's distance north, y, can be determined by;
Cos
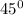 =
=
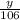
⇒ y = 106 × Cos
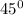
= 74.9533
≅ 75 steps
Musah's distance north from centre to final point is 75 steps.
iv. Musah's distance from centre to final point is AC = AB + BC
= 50 steps + 56 steps
= 106 steps
From ΔACD,
Tan θ =

= 1.0
θ =
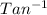 1.0
1.0
=
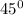
Musah's bearing from centre to final point =
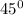 +
+
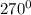
=