Answer:
The equation
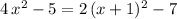
can be solved by first expanding all indicated operations, and later when the constant terms disappear, by factoring out 2x , leaving the equation as a product of two factors equal zero, from which it is easy to extract the roots. See below.
Explanation:
When solving for x in the following expression, and using factoring to apply at the end the zero product theorem:
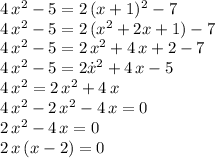
We observe that for the last product, to get a zero, x has to be zero (making the first factor zero), or x has to be "2" making the binomial factor zero.