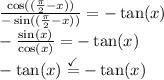Question

Verify the identity. cot(x - pi/2) = -tan(x)

Answer 1

See below.

Explanation:

`\cot(x-(\pi)/(2))=-\tan(x)`

Convert the cotangent to cosine over sine:

`(\cos(x-(\pi)/(2) ))/(\sin(x-(\pi)/(2))) =-\tan(x)`

Use the cofunction identities. The cofunction identities are:

`\sin(x)=\cos((\pi)/(2)-x)\\\cos(x)=\sin((\pi)/(2)-x)`

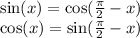
To convert this, factor out a negative one from the cosine and sine.

`(\cos(-((\pi)/(2)-x )))/(\sin(-((\pi)/(2)-x))) =-\tan(x)`

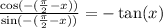
Recall that since cosine is an even function, we can remove the negative. Since sine is an odd function, we can move the negative outside:

`(\cos(((\pi)/(2)-x )))/(-\sin(((\pi)/(2)-x))) =-\tan(x)\\-(\sin(x))/(\cos(x)) =-\tan(x)\\-\tan(x)\stackrel{\checkmark}{=}-\tan(x)`