Answer:

Explanation:
Hello, the Conjugate Roots Theorem states that if a complex number is a zero of real polynomial its conjugate is a zero too. It means that (x-4i)(x+4i) are factors of f(x).
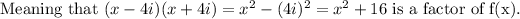
The coefficient of the leading term is 1 and the constant term is -240 = 16 * (-15), so we a re looking for a real number such that.
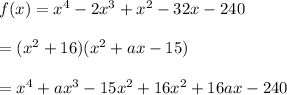
We identify the coefficients for the like terms, it comes
a = -2 and 16a = -32 (which is equivalent). So, we can write in
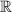 .
.
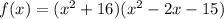
The sum of the zeroes is 2=5-3 and their product is -15=-3*5, so we can factorise by (x-5)(x+3), which gives.
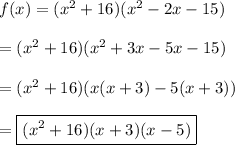
And we can write in
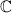
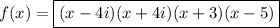
Hope this helps.
Do not hesitate if you need further explanation.
Thank you