Answer:
11.25 amps
Step-by-step explanation:
For transformers, the magnetic flux
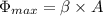
Therefore;
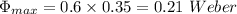
Ф = Фmax (cosωt) = 0.21·(cos(5·t))
From Faraday's law of induction, we have;
ε = -N × dΦ/dt
Which gives;
dΦ/dt = -1.05(sin (5t) )
ε = -N × dΦ/dt = -50× -1.05(sin (5t) )
ε = 52.5(sin (5t) )
I = ε/R = 52.5(sin (5t) )/3.3 = 15.9091(sin (5t) ) amps
The peak current is therefore = 15.9091 amps
The rms current = Peak current /√2 = 15.9091/(√2) = 11.25 amps.