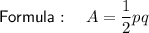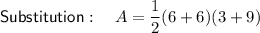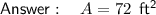Question

Trapezoid area calculations

Answer 1

#1

• 1/2(Sum of parallel sides)Height=Area

Area

• 1/2(6+14)(5.5)
• 1/2(20)(5.5)
• 10(5.5)
• 55in²

#2

Apply Pythagorean theorem

• B²=14²-12²=196-144=52
• B\approx 7

Area

• 1/2(52+8)(12)
• 6(60)
• 360yd²

#3

Area

• 1/2(Diagonals)
• 1/2(6+6)(9+3)
• 1/2(12)12)
• 6(12)
• 72ft²

Answer 2

Formulas Used

`\textsf{Area of a Trapezoid}=(1)/(2)(a+b)h`

where:

• a and b are the bases (parallel sides)
• h is the height (perpendicular to the parallel sides)

`\textsf{Pythagoras' Theorem}: \quad a^2+b^2=c^2`

where:

• a and b are the legs of the right triangle
• c is the hypotenuse (longest side, opposite the right angle)

`\textsf{Area of a Kite}=(1)/(2)pq`

where:

• p and q are the diagonals

-----------------------------------------------------------------------------------------

Question g (Trapezoid)

`\textsf{Formula}: \quad A=(1)/(2)(a+b)h`

`\textsf{Substitution}: \quad A=(1)/(2)(6+14)5.5`

`\textsf{Answer}: \quad A=55\:\: \sf in^2`

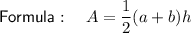
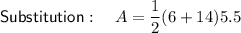
Question h (Trapezoid)

`\textsf{Formula}: \quad A=(1)/(2)(a+b)h`

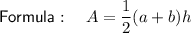
Find the missing side length of the right triangle using Pythagoras' Theorem:

`\implies a=√(14^2-12^2)=√(52)`

Therefore, top edge of trapezoid = √52 + 8

`\textsf{Substitution}: \quad A=(1)/(2)(√(52)+8+8)12`

`\textsf{Answer}: \quad A=96+12√(13)=139.3\:\: \sf yd^2\:(nearest\:tenth)`

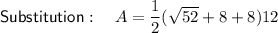
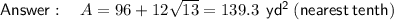
Question i (Kite)

`\textsf{Formula}: \quad A=(1)/(2)pq`

`\textsf{Substitution}: \quad A=(1)/(2)(6+6)(3+9)`

`\textsf{Answer}: \quad A=72\:\: \sf ft^2`