Answer:
63.44 rad/s
Step-by-step explanation:
mass of bullet = 3.3 g = 0.0033 kg
initial velocity of bullet
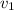 = 250 m/s
= 250 m/s
final velocity of bullet
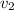 = 140 m/s
= 140 m/s
loss of kinetic energy of the bullet =
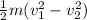
==>
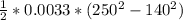 = 70.785 J
= 70.785 J
this energy is given to the stick
The stick has mass = 250 g =0.25 kg
its kinetic energy = 70.785 J
from
KE =
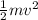
70.785 =
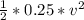
566.28 =

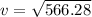 = 23.79 m/s
= 23.79 m/s
the stick is 1.5 m long
this energy is impacted midway between the pivot and one end of the stick, which leaves it with a radius of 1.5/4 = 0.375 m
The angular speed will be
Ω = v/r = 23.79/0.375 = 63.44 rad/s