Question

One positive number is

6 more than twice another. If their product is
1736, find the numbers.

Answer 1

`\Large \boxed{\sf \ \ 28 \ \text{ and } \ 62 \ \ }`

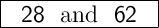
Explanation:

Hello, let's note a and b the two numbers.

We can write that

a = 6 + 2b

ab = 1736

So

`(6+2b)b=1736\\\\ \text{***Subtract 1736*** } <=> 2b^2+6b-1736=0\\\\ \text{***Divide by 2 } <=> b^2+3b-868=0 \\ \\ \text{***factorize*** } <=> b^2 +31b-28b-868=0 \\ \\ <=> b(b+31) -28(b+31)=0 \\ \\ <=> (b+31)(b-28) =0 \\ \\ <=> b = 28 \ \ or \ \ b = -31`

We are looking for positive numbers so the solution is b = 28

and then a = 6 +2*28 = 62

Hope this helps.

Do not hesitate if you need further explanation.

Thank you