Question

Suppose that a household's monthly water bill (in dollars) is a linear function of the amount of water the household uses (in hundreds of cubic feet, HCF).

When graphed, the function gives a line with a slope of 1.55. See the figure below.
If the monthly cost for 15 HCF is $47.84, what is the monthly cost for 9 HCF?

Answer 1

The monthly cost for 9 HCF is $38.54

Explanation:

The graph is missing; however, the question can still be solved

Given

Slope = 1.55

When monthly cost = $47.84, Amount of water = 15 HCF

Required

Determine the monthly cost for 9 HCF

From the question, we understand that it is a linear function;

Linear functions are of the form

`y = mx + b`

Where y is the function of x and m is the slope

In this case; the function is the monthly water bill

and x is the amount of water

Solving for b

When monthly cost = $47.84, Amount of water = 15 HCF

The function is as follows;

`47.84 = 1.55 * 15 + b`

`47.84 = 23.25 + b`

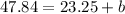
Subtract 23.25 from both sides

`47.84 - 23.25= 23.25 - 23.25 + b`

`24.59 = b`

`b = 24.59`

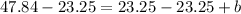
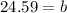
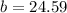
Solving for the monthly cost for 9 HCF

Here, we have that

`x = Amount\ of\ water = 9`

`m = Slope = 1.55`

`b = 24.59`

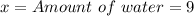
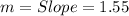
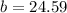
Substitute these values in the linear function;
`y = mx + b`

`y = 1.55 * 9 + 24.59`

`y = 13.95 + 24.59`

`y = 38.54`

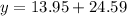
Hence, the monthly cost for 9 HCF is $38.54