Answer:
Answer c): write the function in standard form
Explanation:
To start with, it is important to write the polynomial in standard form, so as to have the two terms with the dependence in x together:
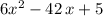
then you extract 6 as a common factor of just the terms with the variable x:
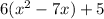
Then proceed to complete the square in the expression inside the parenthesis:
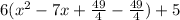
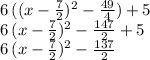
Then, the function can be finally be written as:
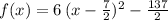
in vertex form