Question

A right triangle has legs with lengths equal to 10 inches and 9x inches. Its hypotenuse measures (x + 10) inches. What is the approximate value of the hypotenuse? 10 inches 10.25 inches 20.25 inches 81 inches

Answer 1

10.25

Explanation:

because I said so ya skoozie

Answer 2

10.25 inches

Explanation:

Given,

Perpendicular ( p ) = 9x

Base ( b ) = 10

Hypotenuse ( h ) = x + 10

Now, let's find the value of x

Using Pythagoras theorem:

`{h}^(2) = {p}^(2) + {b}^(2)`

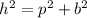
Plug the values

`{(x + 10)}^(2) = {(9x)}^(2) + {(10)}^(2)`

Using
`{(a + b)}^(2) = {a}^(2) + 2ab + {b}^(2)` , expand the expression

`{x}^(2) + 20x + 100 = {(9x)}^(2) + {10}^(2)`

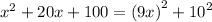
To raise a product to a power , raise each factor to that power

`{x}^(2) + 20x + 100 = 81 {x}^(2) + {10}^(2)`

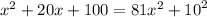
Evaluate the power

`{x}^(2) + 20x + 100 = 81 {x}^(2) + 100`

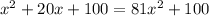
Cancel equal terms on both sides of the equation

`{x}^(2) + 20x = 81 {x}^(2)`

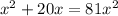
Move x² to R.H.S and change its sign

`20x = 81 {x}^(2) - {x}^(2)`

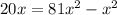
Calculate

`20x = 80 {x}^(2)`

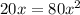
Swap both sides of the equation and cancel both on both sides

`80x = 20`

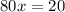
Divide both sides of the equation by 80

`(80x)/(80) = (20)/(80)`

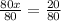
Calculate

`x = (20)/(80)`

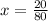
Reduce the numbers with 20

`x = (1)/(4)`

The value of X is
`(1)/(4)`

Now, let's replace the value of x to find the approximate value of hypotenuse

Hypotenuse =
`(1)/(4) + 10`

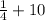
Write all numerators above the common denominator

`(1 + 40)/(4)`

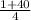
Add the numbers

`(41)/(4)`

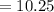`= 10.25` inches

Hope this helps..

best regards!!