Answer:
The correct option is;
0.28
Explanation:
The given data values are;
x, f(x)
8, 12
12, 40
6, 15
20, 20
Where;
x = The number of flowers in the bouquet
f(x) = The total cost (in dollars)
The equation for linear regression is of the form, Y = a + bX
The formula for the intercept, a, and the slope, b, are;
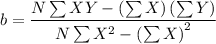
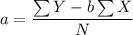
Where:
N = 4
∑XY = 1066
∑X = 46
∑Y = 87
∑X² = 644
(∑X)² = 2116
b = (4*1066 - 46*87)/(4*644 - 2116) = 0.5696
a = (87 - 0.5696*46)/4 = 15.1996
The standard deviation of the x- values

 = 115
= 115
N = 4
Sx =√(115/4)
Sx = 5.36
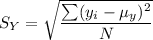
 = 476.75
= 476.75
N = 4
Sy =√(476.75/4)
Sy= 10.92
b = r × Sy/Sx
Where:
r = The correlation coefficient
r = b × Sx/Sy = 0.5696*5.36/10.92 = 0.2796 ≈ 0.28
The correct option is 0.28.