Answer:
m∠C = 90°
Explanation:
Triangle BDC is a right triangle with the measure of angle D = 90°
By applying Cosine rule in the given triangle,
Since, Cosine of any angle in a right triangle is a ratio of Its adjacent side and Hypotenuse (Opposite side of the right angle)
CosC =
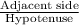
CosC =
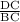
CosC =


C = 28.955
C = 29°
Therefore, m∠C = 29° will be the answer.