Question

Explain why f(x) = x^2-x-6/x^2-9 is not continuous at x = 3.

Answer 1

A: f is not defined at x = -3

Step-by-step explanation: EDGE 2020

Answer 2

See Explanation

Explanation:

Given

`f(x) = (x^2 - x -6)/(x^2 - 9)`

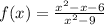
Required

Why is the function not continuous at x = 3

First substitute 3 for x at the denominator

`f(x) = (x^2 - x -6)/(x^2 - 9)`

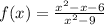
Factorize the numerator and the denominator

`f(x) = (x^2 - 3x+2x -6)/(x^2 - 3^2)`

`f(x) = (x(x - 3)+2(x -3))/((x - 3)(x+3))`

`f(x) = ((x+2)(x - 3))/((x - 3)(x+3))`

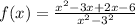
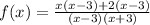
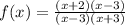
Divide the numerator and denominator by (x - 3)

`f(x) = (x+2)/(x+3)`

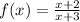
Substitute 3 for x

`f(3) = (3+2)/(3+3)`

`f(3) = (5)/(6)`

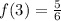
Because
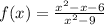`f(x) = (x^2 - x -6)/(x^2 - 9)` is defined when x = 3;

Then the function is continuous