Question

This sequence represents the diameters of circles used to create an art project: 2.5 cm, 3.1 cm, 3.7 cm, 4.3 cm Let f(n) represent diameter in centimeters and n the term number in the sequence. Which equation represents the sequence of diameters? f(n) = 0.6n + 1.9 f(n) = 0.6n + 2.5 f(n + 1) = f(n) + 1.9 f(n + 1) = f(n) – 0.6

Answer 1

f(n) = 0.6n + 1.9

Explanation:

Answer 2

f(n) = 1.9+0.6n

Explanation:

Given the sequence that represents the diameter of a circle

2.5 cm, 3.1 cm, 3.7 cm and 4.3 cm. This sequence forms an arithmetic progression with a common difference.

nth term of an arithmetic progression is expressed as
`T_n = a+(n-1)d`

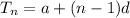
a is the first term of the sequence

n is the number of terms

d is the common difference.

From the sequence above, the first term a = 2.5

common difference = 3.1-2.5 = 3.7-3.1 = 4.3-3.7 = 0.6

Substituting this given values into the formula above will give;

`T_n = 2.5+(n-1)*0.6\\\\T_n = 2.5+0.6n-0.6\\\\T_n = 2.5-0.6+0.6n\\\\T_n = 1.9+0.6n`

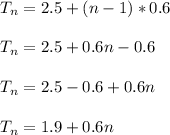
If f(n) represent diameter in centimetres and n the term number in the sequence, the equation that represents the sequence of diameters is

f(n) = 1.9+0.6n