Answer:
Options (2), (3) and (4) are correct.
Explanation:
The given function is f(x) =
![-\sqrt[3]{x}](https://img.qammunity.org/2021/formulas/mathematics/college/rbamxerdi0exwnpne63wtzqpmlkgwzd780.png)
1). First derivative of the function = f'(x) =
![(d)/(dx)(-\sqrt[3]{x} )](https://img.qammunity.org/2021/formulas/mathematics/high-school/enf3fu6f4tawb5tstmmp8bu9g5i5964onu.png)
f'(x) =
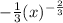
Since derivative is negative, function will be decreasing everywhere.
Therefore, Option (1) is incorrect.
2). Since value of the function is true for every value of all real values of x.
Domain of the function is (-∞, ∞).
Therefore, Option (2) is correct.
3). For the given domain, range of the function will be all real values of y Or (-∞, ∞)
Therefore, Option (3) is correct.
4). Function 'f' when reflected across x axis, equation of the reflected function will be g(x) =
![\sqrt[3]{x}](https://img.qammunity.org/2021/formulas/mathematics/middle-school/ybyl69p7fbrq06x5yarxtkijh1535a0wco.png) .
.
Therefore, Option (4) is correct.
5). For a point (3, -27),
-27 =
![-\sqrt[3]{3}](https://img.qammunity.org/2021/formulas/mathematics/high-school/zkouz702evtdzhpg3p9p6el8p4pjmlnqhf.png)
Therefore, Option (5) is incorrect.