Question

A system of equations is shown on the graph below. How many solutions does this system have?

Answer 1

Hey there!

Only one solution, because they intersect at only one point.

Hope this helps :)

Answer 2

`\boxed{1 \: \: \mathrm{solution}}`

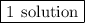
Explanation:

The point where two lines intersect is the solution to the system of equations.

The two lines intersect at (-1, 2).

x = -1

y = 2

y = 2x + 4

y = -x + 1

Plug y as -x+1 in the first equation.

-x + 1 = 2x + 4

-x - 2x = 4 - 1

-3x = 3

x = -1

Plug x as -1 in the second equation.

y = -(-1) + 1

y = 1 + 1

y = 2