Answer:
x=-15/2 and x=1
x=6 and x=3/4
Explanation:
You have the following equations:
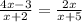
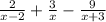
To solve both equations you can first multiply by the m.c.m of the denominators, and then solve for x, just as follow:
first equation:
(x+5)\\\\(4x-3)(x+5)=2x(x+2)\\\\4x(x)+4x(5)-3(x)-3(5)=2x(x)+2x(2)\\\\4x^2+20x-3x-15=2x^2+4x\\\\4x^2-2x^2+20x-3x-4x-15=0\\\\2x^2+13x-15=0](https://img.qammunity.org/2021/formulas/mathematics/high-school/1rpm1tjogb61zj8stjk6x5iun5hs14u3pf.png)
In this case you use the quadratic formula:
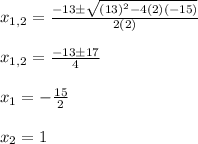
Then, for the first equation the solutions are x=-15/2 and x=1
second equation:
![[(2)/(x-2)+(3)/(x)=(9)/(x+3)]x(x-2)(x+3)\\\\2x(x+3)+3(x-2)(x+3)=9x(x-2)\\\\2x^2+6x+3(x^2+3x-2x-6)=9x^2-18x\\\\2x^2+6x+3(x^2+x-6)=9x^2-18x\\\\2x^2+6x+3x^2+3x-18-9x^2+18x=0\\\\-4x^2+27x-18=0](https://img.qammunity.org/2021/formulas/mathematics/high-school/3v2l3od414cxfv1n9j5mjmbpwsj00ifsly.png)
Again, you use the quadratic formula:
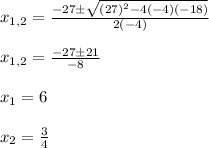
Then, the solutions for the second equation are x=6 and x=3/4