Question

Un lote con forma cuadrada tiene una superficie de LaTeX: \sqrt{\frac{4225}{16}\:\:\:\:m^2}\:\:\:\:\:. Si el dueño del lote quiere colocar 3 hileras de alambres alrededor del terreno, ¿cuantos metros necesitará?

Answer 1

The owner needs 195 meters of wire

Explanation:

If the lot is squared shaped, then its area is given by the formula:

`Area =x^2`

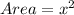
where x is the side of the square. Then considering the value they provide for the surface, each side must be of length:

`x^2=(4225)/(16) \,m^2\\x=\sqrt{(4225)/(16)} \,\,m\\x=16.25\,\,m`

Then the perimeter around this square lot is four times that side length:

Perimeter = 4 (16.25 m) = 65 m

and since the owner wants three rows of wire, the total length of wire needed is:

3 (65 m) = 195 m