Question

Suppose that a wing component on an aircraft is fabricated from an aluminum alloy that has a plane-strain fracture toughness of 26.0 MPa m0.5. It has been determined that fracture results at a stress of 112 MPa when the maximum internal crack length is 8.6 mm. For this same component and alloy, compute the stress level at which fracture will occur for a critical internal crack length of 3.0 mm.

Answer 1

the required stress level at which fracture will occur for a critical internal crack length of 3.0 mm is 189.66 MPa

Step-by-step explanation:

From the given information; the objective is to compute the stress level at which fracture will occur for a critical internal crack length of 3.0 mm.

The Critical Stress for a maximum internal crack can be expressed by the formula:

`\sigma_c = (K_(lc))/(Y √(\pi a))`

`Y= (K_(lc))/(\sigma_c √(\pi a))`

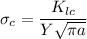
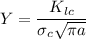
where;

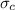`\sigma_c` = critical stress required for initiating crack propagation

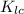`K_(lc)` = plain stress fracture toughness = 26 Mpa

Y = dimensionless parameter

a = length of the internal crack

given that ;

the maximum internal crack length is 8.6 mm

half length of the internal crack will be 8.6 mm/2 = 4.3mm

half length of the internal crack a = 4.3 × 10⁻³ m

From :

`Y= (K_(lc))/(\sigma_c √(\pi a))`

`Y= \frac{26}{112 * \sqrt{\pi * 4.3 * 10 ^(-3)}}`

`Y= (26)/(112 *0.1162275716)`

`Y= (26)/(13.01748802)`

`Y=1.99731315`

`Y \approx 1.997`

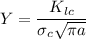
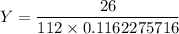
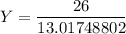
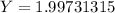
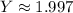
For this same component and alloy, we are to also compute the stress level at which fracture will occur for a critical internal crack length of 3.0 mm.

when the length of the internal crack a = 3mm

half length of the internal crack will be 3.0 mm / 2 = 1.5 mm

half length of the internal crack a =1.5 × 10⁻³ m

From;

`\sigma_c = (K_(lc))/(Y √(\pi a))`

`\sigma_c = \frac{26}{1.997 \sqrt{\pi * 1.5 * 10^(-3)}}`

`\sigma_c = (26)/(0.1370877444)`

`\sigma_c =189.6595506`

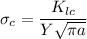
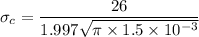
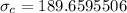
`\sigma_c =` 189.66 MPa

Thus; the required stress level at which fracture will occur for a critical internal crack length of 3.0 mm is 189.66 MPa