Step-by-step explanation:
It is given that,
Wavelength of a wave,
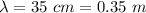
Amplitude,
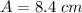
Period of the wavelength, t = 1.2 s
The wave is traveling in a string in the positive x direction. We need to write the wave equation for this conditions.
The general equation of the wave when it is traveling in +x direction is given by :
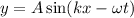
A is amplitude
k is propagation constant
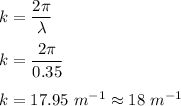
 is angular frequency
is angular frequency
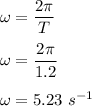
So, the wave equation is given by :
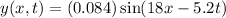
Hence, this is the required solution.