Question

Two terms of an arithmetic sequence are a7 = 32 and a19 =140. . Find n such that Sn = 511

Answer 1

In an arithmetic sequence, consecutive terms differ by a fixed number d :

`a_8=a_7+d`

`a_9=a_8+d=a_7+2d`

`a_(10)=a_9+d=a_7+3d`

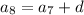
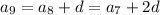
and so on up to

`a_(19)=a_7+12d`

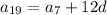
Solve for d :

`140=32+12d\implies12d=108\implies d=9`

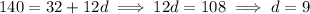
Work backwards to find the first term in the sequence, and hence the n-th term:

`a_6=a_7-d`

`a_5=a_6-d=a_7-2d`

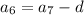
and so on down to

`a_1=a_7-6d`

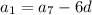
So the first term is

`a_1=32-6\cdot9=-22`

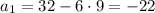
which means the n-th term is

`a_n=a_1+(n-1)d=-22+9(n-1)=9n-31`

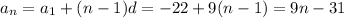
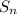`S_n` denotes the n-th partial sum of the sequence, i.e. the sum of the first n terms. We want to find the number of terms such that this sum is 511:

`\displaystyle S_n=\sum_(i=1)^na_i=\sum_(i=1)^n(9i-31)=511`

Distribute the summation and recall the formulas,

`\displaystyle\sum_(i=1)^n1=n`

`\displaystyle\sum_(i=1)^ni=\frac{n(n+1)}2`

`\implies S_n=\displaystyle9\sum_(i=1)^ni-31\sum_(i=1)^n1`

`\implies511=9\cdot\frac{n(n+1)}2-31n`

`\implies1022=9n^2-53n`

`\implies9n^2-53n-1022=0`

`\implies(n-14)(9n+73)=0`

`\implies n=14\text{ or }n=-\frac{73}9`

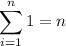
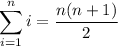
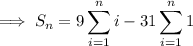
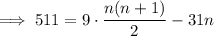
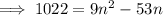
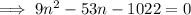
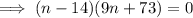
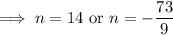
n must be a positive integer, so we the sum is obtained from the first n = 14 terms.