Question

Two cars leave an intersection at the same time. One drives east while the other travels south at 15 miles per hour faster than the other. After 3 hours, the cars are 225 miles apart. How fast is the southbound car driving?

Answer 1

60 mph

Explanation:

Let 'S' be the velocity of the southbound car and 'E' be the velocity of the eastbound car. The distances traveled by each car are:

`D_E=3E\\D_S=3S=3(E+15)\\D_S=3E+45`

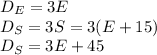
The distance between both cars is given by:

`D^2=D_S^2+D_E^2\\225^2=(3E+45)^2+(3E)^2\\50,625=9E^2+270E+9E^2+2,025\\18E^2+270E-48,600=0\\`

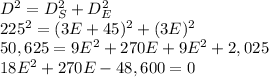
Solving the quadratic equation for the velocity of the eastbound car:

`18E^2+270E-48,600=0\\E^2+15E-2,700\\E=(-15\pm√(15^2-4*1*(-2,700)))/(2)\\E=45.0\ mph`

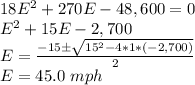
The velocity of the southbound car is:

`S=E+15=45+15\\S=60\ mph`

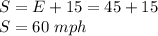
The southbound car is driving at 60 mph.