Question

Grey’s Labs is testing a new growth inhibitor for a certain type of bacteria. The bacteria naturally grows exponentially at a rate of 4.7% each hour. The lab technicians know that the growth inhibitor will make the growth rate of the bacteria less than or equal to its natural growth rate. The current sample contains 90 bacteria. Once a standard tube contains more than 270 bacteria, the sample will stop growing. So, to analyze the effect of the inhibitor over longer spans of time, the lab technicians move the bacteria to larger containers, essentially increasing the container size at a constant rate. This adaptation accommodates 100 more bacteria each hour. The research team wants to track the number of bacteria over time given these two conditions. Select the two inequalities they can use to model this situation.

P ≥ 90e^(0.047t)
P ≤ 270 + 100t
P ≤ 270 – 100t
P ≤ 0.047e^(90t)
P ≤ 90e^(0.047t)

Answer 1

P≤270+100t

P≤90e^(0.047t)

Answer 2

The two inequalities are;

P ≤ 90e^(0.047t)

P ≤ 270 + 100·t

Explanation:

The parameters given for the testing of the new growth inhibitor are;

The growth rate of the bacteria = 4.7% exponentially

The growth inhibitor lowers the growth rate

The population of bacteria after time, t = P

The increase in the number of bacteria per unit time in the 100

The maximum number of bacteria in the standard tube = 270

Therefore, the number of bacteria after the first filling of the tube is P ≤ 270 + 100·t

The equation for exponential growth is
`A_0 e^(kt)`

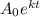
Where:

A₀ = Initial population = 90

k = Percentage growth rate as percentage

t = Time

The equation for the population of bacteria under the influence of the inhibitor is therefore;

P ≤
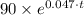`90 * e^(0.047 \cdot t)` which is P ≤ 90e^(0.047t).