Answer:
The change in the volume of a sphere whose radius changes from 40 feet to 40.05 feet is approximately 1005.310 cubic feet.
Explanation:
The volume of the sphere (
 ), measured in cubic feet, is represented by the following formula:
), measured in cubic feet, is represented by the following formula:
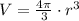
Where
 is the radius of the sphere, measured in feet.
is the radius of the sphere, measured in feet.
The change in volume is obtained by means of definition of total difference:
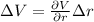
The derivative of the volume as a function of radius is:
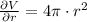
Then, the change in volume is expanded:
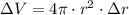
If
 and
and
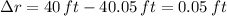 , the change in the volume of the sphere is approximately:
, the change in the volume of the sphere is approximately:
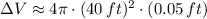
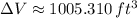
The change in the volume of a sphere whose radius changes from 40 feet to 40.05 feet is approximately 1005.310 cubic feet.