Answer:
2.28% of babies born in the United States having a low birth weight.
Explanation:
The complete question is: In the United States, birth weights of newborn babies are approximately normally distributed with a mean of μ = 3,500 g and a standard deviation of σ = 500 g. What percent of babies born in the United States are classified as having a low birth weight (< 2,500 g)? Explain how you got your answer.
We are given that in the United States, birth weights of newborn babies are approximately normally distributed with a mean of μ = 3,500 g and a standard deviation of σ = 500 g.
Let X = birth weights of newborn babies
The z-score probability distribution for the normal distribution is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean = 3,500 g
= population mean = 3,500 g
 = standard deviation = 500 g
= standard deviation = 500 g
So, X ~ N(
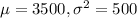 )
)
Now, the percent of babies born in the United States having a low birth weight is given by = P(X < 2500 mg)
P(X < 2500 mg) = P(
 <
<
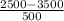 ) = P(Z < -2) = 1 - P(Z
) = P(Z < -2) = 1 - P(Z
 2)
2)
= 1 - 0.97725 = 0.02275 or 2.28%
The above probability is calculated by looking at the value of x = 2 in the z table which has an area of 0.97725.